|
|
GeoAstro Applets |
Astronomy |
Chaos Game |
Java |
Miscel- laneous |
Physics Quiz |
Who is Who ? |
| Start | Saros | Saros-Inex | total eclipses | annular eclipses |
Prediction of Solar Eclipses
|
Eclipses od the Sun are not at all rare: In a calendar year, there are at least 2 and at most 5 solar eclipses. But that maximum of 5 is rarely reached (last: 1935, next: 2206). Of course, not all eclipses are visible from a given place. In modern astronomy the calculation of local circumstances of
a solar eclipse is performed by using the Besselian elements. The Babylonian temple astronomers had been observing the skies and had recorded their observations since the beginning of 2nd millennium BC on clay tablets found in the Mesopotamian region. The earliest total solar eclipse recorded was seen in Ugarit
on Using these data, Babylonian astronomers were able to predict lunar eclipses and -later- solar eclipses with a fair accuracy. They discovered a cycle of 223 intervals between new moons, after which eclipses of the sun and moon recur. The cycle was also known to Thales (*635 BC, famous "eclipse of Thales" in 585 BC), Hipparchos (*190 BC), Pliny (the Elder, * 23 AD), and Ptolemy (*85 AD). In the western word the term saros was uses first by Edmond Halley in 1691. Some of the well known facts are illustrated by my diagrams and tables. Most of the data and details are from the books of Jean Meeus and from the Internet sources listed here. |
Solar Eclipses 1999 to 2024
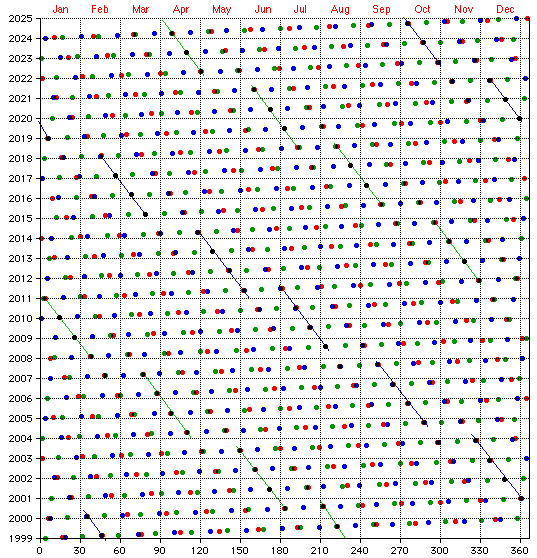
|
|
Solar eclipses have been numbered by van den Bergh (1955):
Diagram showing the Saros numbers
|
An eclipses of the Sun can only take place at New Moon (Sun-Moon conjunction), and only if the Moon is near its orbital node (ascending, or descending). The eclipse zone is about +/- 18.5°, centered on the Moon's node (eclipse of 1935 Jan 05 05:35 UT). The Sun travels along the ecliptic at about 1° per day and requires about 37 days to cross through the eclipse zone centered on each node. New Moon occurs every 29 1/2 days and thus guarantees at least one eclipse during each of the Sun's node crossings. The period during which the Sun is near a node is called an eclipse season and there are two eclipse seasons each year. The saros arises from a natural harmony between three of the Moon's orbital periods: |
Synodic Month new moon to new moon 29.53059 d 29d 12h 44m 6,585.322 d 6,585d 07h 44m 18 years, Draconic Month node to node 27.21222 d 27d 05h 06m 6,585.357 d 6,585d 08h 34m 18 years, Anomalistic Month perigee to perigee 27.55455 d 27d 13h 19m 6,585.537 d 6,585d 12h 53m
(lunation)
10, 11 or 12 days,
and 8 hours
10 or 11 days,
and 8 hours
The diagram is showing the two cases of a solar eclipse:
![]()
![]()
ascending node
descending node
Successive eclipses are generally separated by 6 synodic
months (lunations), a little less than half a year, much rarely by 1 or
5 lunations. Any two eclipses separated by one saros cycle share similar
geometries. They occur at the same node with the Moon at nearly the
same distance from Earth and at the same time of year. Because the
saros period is not equal to a whole number of days, the extra 1/3 day
will shift the central line of the solar eclipse towards the west by
about 120° in geographical longitude. Thus, a Saros series returns
to about the same geographic region of visibility every 3 Saroses
(Triple Saros, exeligmos, 54 years and 31 days). Example:
The total eclipse of 1999, Aug 11 (maximum at 11:03 UT, 45.1N 24.3E, ![]() map) will recur on 2053, Sep 12 (maximum at 09:32
UT, 21.5N 41.8E,
map) will recur on 2053, Sep 12 (maximum at 09:32
UT, 21.5N 41.8E, ![]() map).
map). ![]() World Atlas of Solar Eclipse Paths
World Atlas of Solar Eclipse Paths
Solar Eclipses 1999 to 2024
![]() Eclipse
Home Page (Fred Espenak)
Eclipse
Home Page (Fred Espenak)
|
Type of eclipse: Date / UT / Type / Saros # 1999 Feb 16 06:34 A 140 2000 Feb 05 12:49 P 150 2001 Jun 21 12:04 T 127 2002 Jun 10 23:44 A 137 2003 May 31 04:08 An 147 2004 Apr 19 13:34 P 119 2005 Apr 08 20:36 H 129 2006 Mar 29 10:11 T 139 2007 Mar 19 02:32 P 149 2008 Feb 07 03:55 A 121 2009 Jan 26 07:59 A 131 2010 Jan 15 07:06 A 141 2011 Jan 04 08:51 P 151 2012 May 20 23:53 A 128 2013 May 10 00:25 A 138 2014 Apr 29 06:03 A- 148 2015 Mar 20 09:46 T 120 2016 Mar 09 01:57 T 130 2017 Feb 26 14:53 A 140 2018 Feb 15 20:51 P 150 2019 Jan 06 01:41 P 122 2020 Jun 21 06:40 Am 137 2021 Jun 10 10:42 A 147 2022 Apr 30 20:41 P 119 2023 Apr 20 04:17 AT 129 2024 Apr 08 18:17 T 139 |
A Catalogue of Eclipse Cycles (Robert Harry van Gent)
Predictable Periodic Events - Part II (Jan Curtis)
The Saros Problem (Bruno Kolberg) The Saros
cycles, Eclipse Frequency and Recurrance Jean Meeus:
Mathematical Astronomy Morsels, Willmann-Bell, Second Printing 2000.
Jean Meeus:
More Mathematical Astronomy Morsels, Willmann-Bell, 2002. Jean Meeus:
Mathematical Astronomy Morsels III, Willmann-Bell, 2004. Eclipse: Additional Reading (Encyclopædia Britannica
Online)
From: F. Espenak: Fifty Year Canon of solar eclipses 1986 - 2035, NASA
Ref. Pub # 1178 rev. July 1987
© 2005-2007 Juergen Giesen
![]()